Clifford Algebra, a.k.a. Geometric Algebra, is a most extraordinary synergistic confluence of a diverse range of specialized mathematical fields, each with its own methods and formalisms, all of which find a single unified formalism under Clifford Algebra. It is a unifying language for mathematics, and a revealing language for physics.
Unlike the standard vector analysis whose primitives are scalars and vectors for representing points and lines, Clifford Algebra has additional spatial primitives for representing plane and volume segments in two and three dimensions, and it can be extended to any number of higher dimensions by the same basic scheme, and they do, with remarkably useful properties.
Adding one extra dimension to a total of 4 produces a projective geometry, a concept not exclusive to Clifford Algebra, but very simply expressed in it, with some remarkable invariance properties.
Adding two additional dimensions to a total of 5, enables a conformal geometry with even more extraordinary invariances which are reminiscent of certain properties of perception.
The truth, or validity of Clifford Algebra is confirmed by Occam’s Razor, it provides a simpler model of mathematical objects than does vector algebra, extending naturally from one to two, to three, and higher dimensions all under the same formalism, with a notational economy that simplifies many mathematical expressions.
For example Clifford Algebra reduces Maxwell’s equations of electromagnetism to a SINGLE EQUATION, the rest is implicit in the math!
Another feature of Clifford Algebra is that it uses a coordinate-free representation. Instead of defining motion with respect to an external coordinate system, motion is described with respect to a coordinate frame defined on the object in question, which greatly simplifies many models. Clifford Algebra reveals, for example, that the apparent chirality in electromagnetism, i.e. the right-hand rule for electric generators, and the left-hand rule for electric motors, turns out to be actually an artifact of the math used to describe the world, not a property of the world itself. It turns out that electromagnetism has no chirality, as revealed by Clifford Algebra.
Clifford Algebra fixes many of the problems inherent in linear algebra, from the arbitrary distinction between row-vectors and column-vectors, the complex process of matrix multiplication, the arbitrary arrangement of terms in the matrix, exemplified most clearly by the complexity of the formula for the determinant of a matrix, to determine whether the matrix is invertible. In Clifford Algebra the determinant is the three-dimensional volume of the parallelopiped spanned by the three row vectors of the matrix, and it becomes intuitively obvious that the volume goes to zero when the three vectors are parallel, or when they are all in the same plane. Furthermore, the whole concept of inversion becomes an explicit spatial inversion, a literal turning inside-out of a spatial structure.
Clifford Algebra provides a more intuitive model for subatomic particles that are often modeled with the Pauli matrix, by way of a spatial model that resembles the particle that it models.
And the way that Clifford Algebra achieves this extraordinary Grand Unification of mathematics is by expressing algebraic concepts in the form of spatial operations on spatial structures. Clifford Algebra, a.k.a. Geometric Algebra, is simultaneously a geometrification of algebra, and also an algebrification of geometry. We are accustomed to viewing algebra as a manipulation of abstract symbols back and forth across the equals sign. But do you remember when in geometry, you learned about a transversal crossing a pair of parallel lines, and which angles were similar and which were complementary? Your teacher never had to prove these truths, they merely had to be pointed out, they were self-evident by inspection. Clifford Algebra does that for algebraic manipulations, which become natural and intuitive spatial operations on spatial structures.
Geometry is more primal and explicit than algebra. In Clifford’s own words,
“geometry is the gate of science, and the gate is so low and small that one can only enter it as a child.”
Clifford algebra invites us to stoop low enough to re-examine some of the most basic mathematical concepts that we first learned as children, and to see new spatial and geometrical relations in them.
Why So Obscure?
So if Clifford Algebra is so great, how come you have never heard of it before? In the first place Clifford died at an early age in an era where there were several competing algebras being developed, and Clifford Algebra was eclipsed by Gibb’s Vectors that we all learned in school.
Clifford Algebra was briefly rediscovered by Dirac as a superior expression of his theory of the electron, although the wider significance was not recognized. It was rediscovered again in the 1980’s by David Hestenes who recognized its general applicability and significance, and David has made a careeor of promoting “Geometric Algebra”, a more modern update to the original Clifford Algebra. David Hestenes prefers the term “Geometric Algebra”, which was actually Clifford’s own choice, because “Clifford Algebra” sound like “just another algebra” rather than what it really is, which is a discovery of the fundamental geometrical roots of all of algebra. Besides, Hestenes credits Hermann Grassmann at least equal to Clifford in the development of Geometric Algebra. I use the term “Clifford Algebra” for historical context and honoring a quirky dude, and “Geometric Algebra” when focusing on the modern reformulations and extensions many of which are due to David Hestenes.
My own interest in Clifford Algebra stems from my own conviction that mathematics is not a human invention, but more of a discovery of the foundational principles of thought. Mathematics is an artifact of the human mind and how it represents spatial reality. The properties of mathematics are direct evidence for the principles of operation of the human mind.
And since Clifford Algebra provides such a clear view into the fundamental nature of math, I believe Clifford Algebra to be the Rosetta Stone that reveals the basic computational and representational principles of spatial perception and algebraic cognition.
In fact the many regularities found in mathematics, from the periodicity of the number line, the alternation of even and odd, its mirror symmetry across the origin, and the polarity reversals of negation and inversion, all suggest a phasic / cyclic system of representation not unlike the trace on an oscilloscope in an analog oscillatory circuit, which in turn suggest a wave based representation in the brain underlying both spatial perception and mathematical thought.
Historical Summary
The history of algebra has been a history of the incremental discovery of an apparently pre-existing structure. The natural numbers were discovered independently by all cultures, suggesting that the concept of number is intuitive, or innate. The concepts of zero and the negative numbers seem to follow naturally, as if of necessity, and they too were “rediscovered” independently by different cultures over time.
The closure of circular negation was the first hint of an irregularity.
A problem arose when the solution to certain algebraic equations came out to be equal to the square root of minus one, something that was a mathematical impossibility! Rafael Bombelli, and René Descartes (who coined the term “imaginary” numbers) proposed to name this impossible number i, but that besides the fact that it squares to negative one, in every other way it was a regular number. So for example 1 times i = i. i times i (by definition) = -1. -1 times i must equal -i. And -i times i = -(-1) which brings us back to 1.
 Repeated multiplication by i goes round and round in circles, making periodic excursions into an orthogonal dimension and back again. If you plot the imaginary dimension as a regular spatial dimension, this suggests that multiplication by i corresponds to a 90 degree rotation. This is an example of mathematical closure, a concept we will see again and again in Clifford Algebra.
Repeated multiplication by i goes round and round in circles, making periodic excursions into an orthogonal dimension and back again. If you plot the imaginary dimension as a regular spatial dimension, this suggests that multiplication by i corresponds to a 90 degree rotation. This is an example of mathematical closure, a concept we will see again and again in Clifford Algebra.
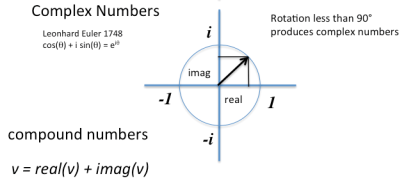
Later it was proposed that you could rotate by less than 90 degrees, which produces a compound number composed of real and imaginary components.
But the real and imaginary dimensions operate by different rules. Multiplication of real numbers scales their magnitudes in or out from the origin. But multiplication of the imaginary component performs a rotation, it is a multiplication that goes round and round instead of in and out.
I don’t know if anyone ever pointed it out to you, but this is VERY STRANGE!
 Josiah Willard Gibbs
Josiah Willard Gibbs
About the same time in the late nineteenth century, Josiah Willard Gibbs was playing around with a different kind of compound number, pairs or triplets of (x,y) or (x,y,z) which can be represented as “directed line segments”, or vectors. The remarkable thing was that you could add or subtract these compound numbers in a manner that made a kind of intuitive spatial sense. The question arose: What happens if you multiply and divide them? Gibbs proposed two kinds of multiplication, the dot product and the cross product, as we all learned in school.
Oliver Heaviside used Gibbs vectors to reformulate Maxwell’s equations of electromagnetism, which did much to cement Gibbs’ vectors position as the dominant paradigm of vector manipulation. Nevertheless, Tait commented that Gibbs’ vectors were “a sort of hermaphrodite monster, compounded of the notations of Hamilton and Grassman”
The dot product was a good idea, and has been incorporated into Clifford Algebra. But it turns out in retrospect that the cross product was a big mistake! In the first place it is only really defined for three dimensions, it does not generalize to two-dimensional vectors without popping out of the page into the third dimension, and in four dimensions the concept is practically meaningless because there are so many orthogonal directions. Furthermore, Gibbs’ vectors can only express scalars and vectors, the only way it could represent a plane is by its surface normal, which is a vector.
Also the cross product can only rotate by 90 degrees, it cannot rotate through intermediate angles as do complex numbers.
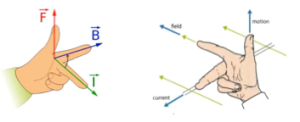 Finally Gibbs’ algebra has a parity problem, the cross product is not preserved under reflection, and thus it introduces a chirality to its model of reality even where there is no chirality in the entity being modeled.
Finally Gibbs’ algebra has a parity problem, the cross product is not preserved under reflection, and thus it introduces a chirality to its model of reality even where there is no chirality in the entity being modeled.
William Rowan Hamilton
In the meantime over in another branch of mathematics, William Hamilton was trying to extend complex numbers, with their elegant rotational multiplication, into three dimensions by proposing three orthogonal imaginary dimensions, i, j,k, each of which behaves like the imaginary dimension i, and they interact with the peculiar circular rule that i x j = k, j x k = i, and k x i = j. Here again we see another example of mathematical closure, a curious property which turns out to be very significant.
But Hamilton ran into a problem defining division for these imaginary vectors because the quotient was not unique. Imaginary vectors A and B, each composed of (i,j,k) components, multiply meaningfully as in A x B = C, but the inverse, C / B does not produce a single unique value but a whole range of possible values. This algebra lacked closure, it only computes one way, you cannot calculate backwards, as in moving terms back and forth across the equals sign.
After many years of frustration, in a flash of intuition (immortalized by a plaque on the Broom Bridge in Dublin where the intuition first struck him) Hamilton realized that he needed one more scalar value to provide a scale for his complex vectors, so he defined a compound number of the form v = a + bi + cj + dk, which could be both multiplied and divided, and the quaternion was born!
All this occurred in the era when the theory of relativity was first emerging, and 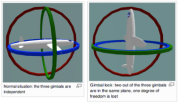 Hamilton incorporated a relativistic concept in his quaternions by expressing rotations not with reference to a global x,y,z coordinate frame, but relative to the current orientation. For example if the orientation of an aircraft in flight is expressed in “Euler angles” as a triplet of roll, pitch, and yaw angles in absolute coordinates, there is a problem known as “gimbal lock” when the aircraft is heading either straight up, or straight down, where it has no defined yaw angle, or heading. Instead, quaternions employ a coordinate system that rotates with the aircraft, as perceived by the pilot in the aircraft, where concepts of “pull up”, “roll left”, and “yaw right” are meaningful whatever the current orientation of the aircraft.
Hamilton incorporated a relativistic concept in his quaternions by expressing rotations not with reference to a global x,y,z coordinate frame, but relative to the current orientation. For example if the orientation of an aircraft in flight is expressed in “Euler angles” as a triplet of roll, pitch, and yaw angles in absolute coordinates, there is a problem known as “gimbal lock” when the aircraft is heading either straight up, or straight down, where it has no defined yaw angle, or heading. Instead, quaternions employ a coordinate system that rotates with the aircraft, as perceived by the pilot in the aircraft, where concepts of “pull up”, “roll left”, and “yaw right” are meaningful whatever the current orientation of the aircraft.
Hamilton introduced another innovative departure from conventional mathematics by proposing a  multiplication which was not commutative, i.e. that a x b is not equal to b x a, but neither are they unrelated, but in fact a x b = -(b x a), where negation corresponds to a reversal in the direction of rotation, just as negation of a real vector reverses its orientation. These intriguing concepts were incorporated into Clifford Algebra.
multiplication which was not commutative, i.e. that a x b is not equal to b x a, but neither are they unrelated, but in fact a x b = -(b x a), where negation corresponds to a reversal in the direction of rotation, just as negation of a real vector reverses its orientation. These intriguing concepts were incorporated into Clifford Algebra.
Closure
Now a word on the concept of closure, which will turn out to be significant throughout this presentation. In algebra closure refers to operations on an algebra that remain within the algebra. For example the natural numbers are closed under addition because the sum of any two natural numbers is also a natural number, but they are not closed under subtraction, because if you subtract enough you can fall right off the end of the natural number scale.
Likewise, the integers are closed under addition, subtraction, and multiplication, but they are not closed under division because certain quotients fall between the cracks of the integers. But that is not the only kind of closure in math.
0 1 2 3 4 5 6 7 8 9
There is closure in our numeral system, where when we run out of digits, we just put a zero and carry to the next column.
There is closure in an odometer where each wheel flips from 9 to 0, and the whole register flips from 99999 +1 to 00000.
There is closure in digital registers that also flip from FFFF +1 to 0000.
It’s a computational principle that prevents the calculation from “running off the edge of the page”, or the register, allowing infinite calculation within a finite representation.
And there is closure in a multiplication that goes round and round instead of in and out. It defines a continuous space like a section of the x axis, but it is a closed dimension, that is finite, but boundless. This will turn out to be very significant.
Hermann Günther Grassmann
 Meantime Hermann Grassmann, the inventor of linear algebra, proposed an alternative to Gibbs’ flawed cross product, in the form of the exterior product, or wedge product, whereby the wedge product of two vectors defines a patch of surface whose area is equal to the product of the two vectors, as if one vector was “swept” along the other (or the other “swept” along the first), and that surface is within the plane that contains both of the original vectors. Just as a vector has magnitude and orientation, but it has no defined location because it is always at the “origin”, in the same way, the bivector, or wedge product of two vectors has an orientation and an area, but it has no location, and neither does it have a shape. It is an oriented area.
Meantime Hermann Grassmann, the inventor of linear algebra, proposed an alternative to Gibbs’ flawed cross product, in the form of the exterior product, or wedge product, whereby the wedge product of two vectors defines a patch of surface whose area is equal to the product of the two vectors, as if one vector was “swept” along the other (or the other “swept” along the first), and that surface is within the plane that contains both of the original vectors. Just as a vector has magnitude and orientation, but it has no defined location because it is always at the “origin”, in the same way, the bivector, or wedge product of two vectors has an orientation and an area, but it has no location, and neither does it have a shape. It is an oriented area.
These bivectors can be added and subtracted just like regular vectors, and if a bivector is multiplied  by a vector, its surface is “swept” along that vector in the same manner to define the volume spanned by the three component vectors, an entity known as a trivector. In other words, this is an algebra that generalizes to higher dimensions by the same exact principles that apply at the lower dimensions, and this provides an algebraic entity for scalars, vectors, bivectors, trivectors, and there is no limit to the number of dimensions it can be extended to.
by a vector, its surface is “swept” along that vector in the same manner to define the volume spanned by the three component vectors, an entity known as a trivector. In other words, this is an algebra that generalizes to higher dimensions by the same exact principles that apply at the lower dimensions, and this provides an algebraic entity for scalars, vectors, bivectors, trivectors, and there is no limit to the number of dimensions it can be extended to.
Like Hamilton’s quaternions, Grassman’s wedge product is anti-commutative, whereby a^b = -(b^ a). And it also incorporated a kind of closure whereby (in three dimensions) a^b^c makes a trivector, but a^b^c^d collapses back down to a scalar so as to prevent runaway expansion into ever higher dimensions.
a). And it also incorporated a kind of closure whereby (in three dimensions) a^b^c makes a trivector, but a^b^c^d collapses back down to a scalar so as to prevent runaway expansion into ever higher dimensions.
William Kingdon Clifford
William Kingdon Clifford was the man who took all the best ideas from previous work to achieve a single consistent formalism that generalizes to arbitrary numbers of dimensions. Clifford defined a hierarchy of compound number knows as “clifs”, or “multivectors”, that range from zero dimensions (scalar) to one dimension (vector) to two dimensions (bivector), to three (trivector), and on upward as far as you care to go. In Clifford Algebra dimensions are referred to as “grades”, so a scalar is grade zero, a vector is grade 1, etc.
In order to attain the kind of closure seen in quaternions, you have to decide from the outset how many dimensions you want to use for your problem, so for example two-dimensional Clifford algebra, or Cl2 wraps around at two dimensions so as not to protrude out of the plane into the third, whereas Cl3 has closure in three dimensions, so as not to protrude into the fourth.
Just as a real number can be considered as a complex number with zero imaginary component, a clif  can be considered to be composed of higher order clifs up to the grade of the algebra you choose, even if many of them happen to have zero coefficient for any particular problem. Clifford Algebra of zero dimensions, Cl0, is just regular scalar algebra. Clifford Algebra of one dimension, Cl1, is composed of a scalar and a vector. Cl2 is composed of a scalar, two vectors, and a bivector. Cl3 is composed of a scalar, three vectors, three bivectors, and a trivector, and that same scheme extends upward to higher dimensions by the same principle.
can be considered to be composed of higher order clifs up to the grade of the algebra you choose, even if many of them happen to have zero coefficient for any particular problem. Clifford Algebra of zero dimensions, Cl0, is just regular scalar algebra. Clifford Algebra of one dimension, Cl1, is composed of a scalar and a vector. Cl2 is composed of a scalar, two vectors, and a bivector. Cl3 is composed of a scalar, three vectors, three bivectors, and a trivector, and that same scheme extends upward to higher dimensions by the same principle.
Here we see the hierarchy of Clifford Algebras each with its own characteristic pattern of components, that appear in numbers corresponding to Pascal’s triangle. Two-dimensional clifs, Cl2 (the even sub-algebra) are mathematically isomorphic to complex numbers. Three-dimensional clifs, Cl3 are isomorphic to Pauli matrices used to model subatomic particles. Cl4 is isomorphic to Dirac matrices that model relativistic subatomic particles.
Pauli matrices used to model subatomic particles. Cl4 is isomorphic to Dirac matrices that model relativistic subatomic particles.
Lets take a closer look at Cl3, Clifford Algebra in three dimensions. There is a scalar, e, then three orthogonal basis vectors e1, e2, e3, then three bivectors obtained by pairwise multiplication of the basis vectors, and finally a trivector defined by the wedge product of all three basis vectors. Each bivector has a kind of twist that represents a rotation by 90 degrees, for example bivector e1^e2 represents the right-angled turn from the direction e1 to e2, while its negation, -(e1^e2) rotates by the same angle but in the opposite direction.
Multiplication of all three basis vectors (e1^e2^e3) produces a three-dimensional volume segment or trivector, with unit cubed volume. This trivector has some peculiar properties that represent the scale of the whole space, it is often called the pseudoscalar of the space, I, it performs the function of the imaginary number i, but extended into three dimensions. Multiplication by the trivector represents a 90 degree turn in all three dimensions. Therefore two such 90 degree turns in each of the three dimensions results in a total reversal of direction, which is equivalent to a simple negation, and thus I**2 = -1. Clifford Algebra eliminates the mystery of the concept of the imaginary number, which becomes a simple rotation by 90 degrees.
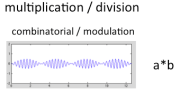
For all the variety of different clifs of their various grades, there are basically two ways that clifs can interact, one is addition/subtraction (add-traction?) and the other is multiplication/division (multipli-vision?). Addition represents a kind of summation of independent or successive processes, whereas multiplication represents a simultaneous modulation of one clif by another; if either clif is negative the product is negative, but if both are negative the product is positive again, another manifestation of closure. In fact analog modulation as in AM radio, or linear gain in amplification, is defined exactly as analog multiplication.
These two very basic and primal operations can be applied between clifs of any grade, and they can be applied across different grades, and in every case they produce results that seem intuitively consistent and reasonable as one might expect. Addition of scalars and vectors produces a compound scalar and vector product. Addition of vectors proceeds logically as in Gibbs’ vectors. Bivectors add to other bivectors to produce a sum bivector.
A scalar times a vector scales the vector in length, and reverses its direction if negative. A vector times a vector makes a bivector with a twist that turns from the direction of one to that of the other. A scalar times a bivector scales its area. A vector times a bivector, or the product of three vectors produces a trivector. A vector times a trivector does not expand to a quadrivector, but rather it collapses back down to a scalar, thus maintaining closure in three dimensions.
There is an interesting interaction that occurs across grades seen for example when a scalar, a non-spatial entity, multiplies a vector which has linear extent, it changes the spatial length by the magnitude determined by the scalar value. Likewise, taking the magnitude of a vector v as in ||v|| summarizes the spatial extent of that spatial vector with a non-spatial scalar magnitude. A bivector B can also be reduced to a scalar magnitude by taking its magnitude ||B||, which provides an abstract measure of the magnitude of the bivector which is proportional to its area. These are manifestations of inter-grade communication, whereby the scalar value can be seen as the most abstract representation, reducing clifs to a single scalar value that records their magnitude, and thus scalar algebra can be seen as an abstraction of the principles of vector algebra, preserving the corresponding magnitudes but discarding spatial extent.
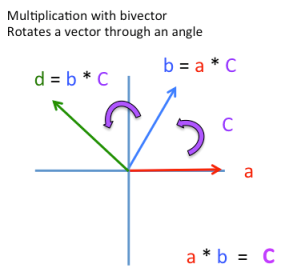
Lets take a closer look at the product of two vectors. If the two vectors are parallel, the product collapses to a scalar, yet another manifestation of closure. A vector times itself collapses to a scalar value one, meaning the vector is a perfect match to itself. If the vectors are pointing in different directions, the product includes a bivector with a twist proportional to the area spanned by the two vectors.
 We can see the effects of the twist by multiplying a different vector by the bivector, which rotates the vector through the same angle as that between the original vectors. Vector a times bivector c (= a x b) rotates a through the angle from a to b, which leaves it equal to b. Vector b times bivector c produces d, rotated from b through the same angle as that between a and b. As with quaternions,
We can see the effects of the twist by multiplying a different vector by the bivector, which rotates the vector through the same angle as that between the original vectors. Vector a times bivector c (= a x b) rotates a through the angle from a to b, which leaves it equal to b. Vector b times bivector c produces d, rotated from b through the same angle as that between a and b. As with quaternions,
Since Clifford multiplication is anti-commutative, it is important to distinguish a*b from b*a, depending on which is the pre-multiplier and which the post-multiplier. For example a*b is a rotation from a to b, when applied to a, as in a*(a*b) it rotates a into b, or b = a*(a*b). But the term can be grouped differently by moving the parentheses, i.e. (a*a)*b. Since a*a = 1, (a*a)*b = b, which produces the same result, so everything works out right in the end.
The Geometric Product
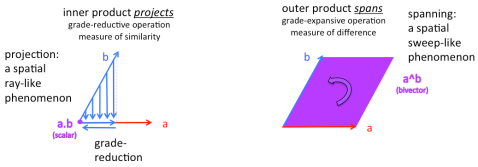
As with Gibbs’ vectors with its distinction between the dot and cross products, Clifford multiplication, known as the geometric product, is actually a compound multiplication composed of the sum of a dot product and a wedge product. Like Gibbs’ vectors, this product captures two aspects of multiplication. The dot product is a measure of similarity between two vectors, and is a scalar quantity, whereas the wedge product is a measure of difference, or what you’d have to do to one to transform it into the other. These are complementary operations, each one capturing what the other misses. Significantly, both are spatial projective operations, one is grade-reducing projection, the other is grade-expanding sweep. The inner product projects one vector onto the other like casting a shadow, although the shadow is then projected again to a scalar magnitude. The outer product spans the space between the vectors, a spatial sweep-like operation that is grade-increasing.
These two operations are not just complementary by design, but by definition. The dot product is computed as the (average of the) product, a*b, added to its reverse b*a. Adding a product to its reverse cancels out any component that is asymmetrical, i.e. the clockwise twist cancels its counter-clockwise reverse, leaving only the symmetrical component, which is the dot product, or what the two vectors share in common. It is a little peculiar to call this operation a “multiplication”, because multiplication is generally thought of as producing a multiplicity of its multiplicands; it is generally an expansive operation (unless the multiplicands are less than one), whereas the dot product is more like a collapse to lower grade, more akin to what we think of as division.

The wedge product is defined as the (average of the) difference between the product and its reverse, an operation that eliminates the symmetrical component, but the reversal of the reverse by negation makes them both rotate in the same direction, whose average preserves only the asymmetrical component, that represents the difference between the vectors, or how one is twisted from the other. The dot and wedge products are therefore like symmetry filters that filter the symmetrical and asymmetrical components of their multiplicands, respectively.
 A (scalar ^ a vector) makes a vector. A (vector ^ a vector) makes a bivector. A (bivector ^ a vector) makes a trivector. The wedge product is a grade-increasing operation. A trivector dot a vector collapses the dotted dimension. Trivector (P^Q^R) . R collapses the R dimension to make bivector P^Q. Bivector (P^Q) . Q collapses to P. Vector P dot P collapses to 1. The dot product therefore seems more akin to division than to multiplication.
A (scalar ^ a vector) makes a vector. A (vector ^ a vector) makes a bivector. A (bivector ^ a vector) makes a trivector. The wedge product is a grade-increasing operation. A trivector dot a vector collapses the dotted dimension. Trivector (P^Q^R) . R collapses the R dimension to make bivector P^Q. Bivector (P^Q) . Q collapses to P. Vector P dot P collapses to 1. The dot product therefore seems more akin to division than to multiplication.
It is interesting to note that a scalar * scalar * scalar * scalar is still a scalar! You need to have at least one spatial dimension to start expanding in grade. The basis vectors e1 e2 e3 can be thought of as pre-existing “generators” capable of generating a vector as soon as they are supplied with a non-zero coefficient.
Here is an animation of the product of the two red vectors as one rotates around the other, showing the complementarity of the dot and wedge products. (The dot product is depicted here as a vertical vector, to show its magnitude, although it is actually supposed to be a scalar quantity) Note the several polarity reversals of both the dot and wedge products as as the vectors go around, along with a reversal in the rotational orientation, or “twist” of the bivector.
Clifford Vectors Analog Electronics Analogy
This series of phase reversals, and the concept of a generator, is highly suggestive of a cyclic phasic representation not unlike the trace on an oscilloscope, as demonstrated in the above op-amp circuit. A sawtooth waveform generator at the upper-left generates the sawtooth wave plotted on the lower left, which is split into x and y streams each of which can be modulated by the potentiometers toward the right to produce X and Y traces whose amplitude can be modulated (and reversed) with the X and Y sliders to the right, plotted at the lower center of the screen. The X and Y signals are then plotted against each other at the lower right, showing a “flying spot” starting at the origin and flying cyclically toward the upper-right, tracing out the vector represented by the oscillations in the system. If you reverse the X slider the vector rotates into the second quadrant. If you then reverse the Y slider the vector rotates into the third quadrant, if you reverse the X slider back to positive the vector rotates into the fourth quadrant, and if you bring Y back to positive the vector returns to the first quadrant. The sawtooth waves that control the “flying spot” that traces out the vector correspond to the basis vectors e1 and e2, which are constantly cycling, but only produce a trace when their coefficient is non-zero.
The basic principles behind Clifford Algebra appear rather simple, which makes it all the more wonderous what complex computations they can achieve.
Clifford Algebra has been characterized as a “reflection algebra” because its most primal operation, vector multiplication, seems to mimic a simple reflection. If red vector c = b*a*b is the product of b times (a*b), it is a reflection of a through b, because the angle from c to b is the same as the angle from b to a. (We are dealing here with unit vectors so as to avoid any additional scaling, resulting in pure rotation)
This Clifford Vector Multiplication Animation shows how the reflection of a in b occurs like a reflection in a plane surface normal to vector b.
The rich and powerful operation of that rotational Clifford multiplication can be reduced to a simple reflection!
This rotational multiplication by reflection is reminiscent of a phenomenon in nonlinear optics when two laser beams cross in space, as shown for beams B1 and B2 above. An interference pattern appears through the zone of their intersection due to constructive and destructive interference. If modeled in a Reciprocal Lattice representation where each beam is represented by a vector, as shown by K1 and K2 in C above, the emerging interference pattern can itself be described as the difference vector K2 – K1 that closes the wave vector diagram.
If the crossing occurs in empty space it has no effect on anything else. However if the crossing takes place in the transparent volume of a nonlinear optical materal (and most any glass goes nonlinear with sufficient amplitude) the interference pattern warps the glass in the shape of the pattern, by the optical Kerr effect, which defines parallel planes of alternating refractive index in the glass.
This reified interference pattern in turn is able to deflect, or reflect a third beam, B3 above, producing a fourth reflected beam B4 whose direction and magnitude can also be computed as the vector that closes the wave vector diagram in the reciprocal lattice representation in B above, i.e. such that K1+K2+K3+K4 = 0. This is the principle behind phase conjugation, a mechanism by which the angle between two vectors controls the reflection of a third, reminiscent of the bivector and its effect on vectors that are multiplied with it.
Maxwell’s Equations in Clifford Algebra
The power of the geometric product is demonstrated most dramatically in the example of Maxwell’s four Equations of Electromagnetism. These describe the curl of electric and magnetic fields using cross-product terms, and the divergence of the electric and magnetic fields using dot product terms.
The magnetic field must be described by a “pseudo-vector” field because unlike a plain vector, as with the electric field, the magnetic field cannot survive an “improper rotation” i.e. a reflection without requiring an additional change of sign. (The mirror image of your right hand demonstrating the right-hand rule is your left hand, but that demonstrates a left-hand rule!)
In Clifford Algebra these four equations reduce to a single equation, the rest is implicit in the math! The magnetic field is described by a bivector, which has the sign-flipping automatically built-in. The geometric product product automatically multiplies the wedge product terms for the curl, and the dot product terms for divergence, all in a single equation.
Algebraic / Spatial Concepts
There are a number of algebraic concepts, some of which are familiar in scalar algebra, that are revealed by Clifford Algebra to be actually algebraic / spatial concepts. For example the reflection of a vector about the origin is equivalent to negation. Reflection is negation! Clifford Algebra is a reflection algebra. Rotation by 180 degrees is also a negation! Rotation by 90 degrees is equivalent to multiplication by i, the square root of minus one, because two of those multiplications reverse the direction which is also negation. This removes the mystery behind that peculiar notion of the square root of minus one, and reveals it to be a simple rotation. Furthermore, negation is also rotational reversal, thus drawing an equivalence between the open translational dimensions and the closed circular dimensions. This is very strange! Its profound significance will be discussed below.
This is simultaneously an algebrification of geometry, and the geometrification of algebra, it suggest a fundamentally spatial computational mechanism behind the principles of algebra. The similarity between numbers arrayed along the number line, and the lengths of corresponding vectors from the origin, is no mere coincidence, it reveals spatial vectors as the true basis underlying even scalar algebra.
It is a peculiar characteristic of Clifford Algebra that angles are not represented directly as angles, expressed in degrees or radians, but rather, angles are expressed by their X and Y components, a rectilinear coordinate system for expressing angles. This is significant because it makes a connection between the egocentric “dartboard” polar coordinate system where the origin is a special unique place, and the allocentric “city-block” Cartesian coordinate system in which every point is identical, and the origin is no longer special. Clifford algebra expresses one in terms of the other, thus mapping them to each other.
Another curious algebraic / spatial concept is the Dual, indicated by a superscript* asterisk. Every Clifford Algebra entity has its “orthogonal complement”, like the particle and its antiparticle, or the yin and its yang. For example the dual of a vector is a bivector, the wedge product of a pair of vectors that are orthogonal to it, and the dual of the bivector is its normal vector. The area of the bivector that is the dual of a vector is proportional to the magnitude of the vector.
The dual of a scalar, which has no spatial extent, is the pseudoscalar, which has all the spatial extent, and the dual of the pseudoscalar is a scalar.
The dual of a clif is easy to calculate, you just divide by the pseudoscalar, and this is a consequence of closure, because a vector times itself collapses to a scalar, so multiplying by the pseudoscalar collapses all vectors that are non-zero, leaving only those that were zero to begin with. It flips the whole pattern into its opposite, and the opposite of the opposite is back again to the apposite, the thing you began with in the first place.
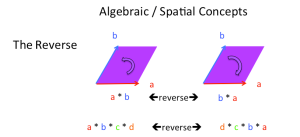
Another algebraic / spatial concept is the reverse. For example the reverse of a*b is b*a. The reverse of a*b*c*d is d*c*b*a. Its like multiplying yourself back out again in the reverse sequence than the way you got in. We have already seen the utility of the reverse in defining the dot product and the wedge product by symmetry and anti-symmetry respectively.
Another interesting concept is the Nullspace, defined for a Clif as the set of all vectors that evaluate to zero for some function. For example the Inner Product Nullspace (IPNS) of a vector v is the set of all vectors whose dot product with v = 0, which consists of the plane that is orthogonal to v. This is in contrast to the dual of that same vector, which is a bivector normal to the vector, i.e. within the plane of the IPNS, but with an area proportional to the length of the vector, whereas the IPNS of a vector is a plane that extends to infinity. This is a generalization of the outer product to infinity. (Note that the NULL in the nullspace is a negation, thus the Inner Product NullSpace resembles an OUTER product, while the Outer Product NullSpace resembles an INNER product)
The Outer Product Nullspace (OPNS) of a vector is the set of vectors whose outer product is zero, i.e. the infinite line within which the finite vector is embedded. Again, this contrasts with the dual of a bivector which is a vector whose length is proportional to the area, whereas the OPNS of a bivector is a line that stretches to infinity in opposite directions.
These are all examples of the principle of reification, extrapolating a given local pattern outward to infinity by the same rules. A vector expands to an infinite line, a bivector surface segment expands to an infinite plane. It is easy enough to comprehend and to describe this kind of reification, but very hard to implement in any kind of finite mechanism, except by a parallel analog spatial principle like an optical mirror system.
Projections are so fundamental to Clifford Algebra that they can be expressed very simply using dot and wedge products.
For example a vector a can be decomposed into parallel and perpendicular components to a plane defined by bivector B, where the parallel component, “a par B” = a.B/B, whereas the perpendicular component “a perp B” = a^B/B. David Hestenes calls this powerful pair of complementary concepts “projection” and “rejection”.
We have seen how reflections can be performed by multiplication. Reflections can also be computed from simple projections. For example the reflection of vector a in bivector U above can be calculated as the component parallel to the plane minus the component perpendicular to it, i.e. “par – perp”.
Rotation by reflection produces a mirror-image reversal in the reflection, as does a normal mirror. This is not readily apparent in the case of vectors due to their one-dimensional symmetry, but is of significance for higher order clifs.
A more general concept of rotation is derived by two reflections through two vectors, which flips the reflection back again, resulting in a rotation without flipping. For example vector “bab” which is (b*a*b) is a reflection of vector a through b, and similarly, the reflection of bab on vector c is computed as c*bab*c, a double reflection “sandwich”
This concept extends naturally into 3-D. For example vector a is reflected through n by the rotation n*a*n, (start with n, then rotate through the angle from a to n) and then that vector “nan” is reflected through another vector m, resulting in a final product of m*nan*m, or m*n*a*n*m.
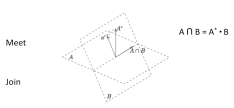
Another algebraic / spatial concept is the concept of the “meet” and the “join” between two Clifs corresponds to the Intersection and Union of the component entities. These too have simple formulas in Clifford Algebra.
The Inverse Function
Now we come to the concept of inversion, 1/x, a concept you learned so long ago that you probably can’t even remember learning it, but in all the time since then, nobody ever pointed out to you that it is a conceptual impossibility! Supposedly for every value x that can range from one to INFINITY, there is a reciprocal value 1/x that is confined to the interval between zero and one. That suggests a one-to-one mapping from an infinite range to a finite range between zero and unity!
This is demonstrated here by plotting in red the reciprocal function between zero and one, i.e. the number whose inverse maps to it. For example ½ maps to 2, 1/3 maps to 3, ¼ maps to 4, and so forth, showing how the inverse function (the red plot line between zero and one) is like a conformal reflection of the number line (the black line plotted from one towards infinity) reflected through the unit boundary although squashed by a conformal mapping.
Its as if you took the number line from one to infinity, made a mirror-image reflection of it, take the reciprocal of that, Then we effectively put a bracket BEYOND INFINITY (the arrow coming in from the left) and squash the reflection back down to a finite range so that the entire reflection is squashed into the unit interval. This is a completely impossible operation because it pretends that it is possible to place a bracket beyond infinity and to squash infinity back to “finity”, a finite range. And on our familiar number line the same operation is done in the negative direction too. This creates a double mirror image of the whole number line from negative to positive infinity excluding the unit radius, all packed within the finite range of the unit radius from -1 to +1. This is an extraordinary paradoxical impossibility, but it is the impossibility that brings closure to multiplication, that offers a reciprocal to multiplication as if every positive and negative number had a reciprocal value that falls between positive and negative unity, and the fact that every number has its reciprocal is what makes it possible to multiply and divide both sides across the equals sign, to calculate forward to compute implications, as well as backward to impute original causes.
This incredible “impossible” reciprocal function was already present in scalar algebra that we learned in grade school. What Clifford Algebra adds to this concept is a circular symmetry, like a set of number lines rotated through all orientations, tracing a unit-radius circle at the center, within which is a conformal reflection of the infinite surrounding space. For every (x,y) point in two-dimensional space that falls on some radial ray from the origin, there is a reciprocal point that falls on the same ray in the same direction from the origin, but with radial distance from the origin that is the reciprocal of the distance to the point, just as occurs on the regular number line itself. If the original point is greater than unity, then its reciprocal is always less than unity, and vice-versa, because by symmetry, the reciprocal of a reciprocal gets back to itself. Clifford Algebra generalizes the algebra on the familiar number line to an algebra that occurs symmetrically at all orientations equally, and thus merges algebra with its parent geometry to define an algebra of space. And this same concept extends naturally into three dimensions and higher, where the central unit-radius sphere, or hyper-sphere, contains within it a reciprocal inverse conformal reflection of the entire space outside the sphere squashed into a finite spherical volume.
Play around with MathForum.org‘s Inversion of A Point Demo and observe that when point x and its inverse point 1/x are close to the circumference of the unit circle, they are mirror-reflections of each other across the unit circle, showing how multiplication is reflection near the unit boundary. When you slide point x off outward towards infinity, its reciprocal point 1/x begins to approach zero, but never gets there unless you could slide x all the way out to infinity! Now if you slide x back from infinity and make it cross the unit circle, it changes place with its reciprocal doppelgänger like Alice changing places with her own reflection in a mirror, and now as x approaches the central origin, its reciprocal shoots off towards infinity. The difference this time is that you can move point x exactly to zero, which means that its reciprocal must have arrived at actual infinity, although no computer display could possibly show it there, we just stop accounting for it when it runs off the edge of our screen.
Alexandre Duret -Stereographic projection
Alexandre Duret’s photo art Stereographic Projection gives an impression of how this idea would look extend into three dimensions, with a spherical boundary at a unit distance from some origin, containing an inverse conformal reflection of the surrounding world. See the tall tower at 2 o’clock, blocking the sun, and its inverse reflection within the unit radius in the 2 o’clock direction, with a peculiar inversion: the tower itself extends outward from the center above its base (“up” is “outward”). In the reflection the tower extends inward from the base toward the center, (i.e. “up” is now “inward”) because in the inverse world, the direction towards the circumference of the circle is proximal, whereas the direction towards the center of the circle is distal, with the bizarre peculiarity that the singular point at the very center of the picture represents infinity in all directions! This is a very strange singular structure to be found at the very core of our number system! It is not there by accident. It provides a lever balance across the unit boundary between a value x and its reciprocal 1/x.
The inverse function embodies an assumption that all of infinity in every direction can be packaged as its reciprocal within a finite unitary range, the finite range from zero to one reflects the entire world from one to infinity. And even more outrageous to common sense, is the fact that although every direction in space points outward towards its own unique infinity in that direction, in the reciprocal reflection they all map to a single central point, which represents not only infinity, but all of the infinities in all directions simultaneously!
In my next post I will show how the impossibility of the inverse function suggests that the number line should not be conceived as infinite, but merely a pseudo-infinity that represents the entire range from one to infinity, all squashed into a finite range, a mirror-image of its conformal reflection, as suggested in non-euclidean geometries.
And of course the same thing would be required in the negative direction. That would render the point-for-point mapping between x and its inverse 1/x no longer an impossible paradox, but now perfectly possible, because it is now a point-for-point mapping from a finite range to another finite range. The existence of the paradox of the inverse function is evidence that our true representation of mathematics in our mind does not use a representation that extends to infinity (how could it?) but it simulates infinity within a finite representation that does not incorporate a paradoxical impossibility.
In fact this much should have been obvious by inspection of our world of perceptual experience, which itself seems to be trapped in some kind of egocentric warp. The sides of a long straight road converge to a point on the horizon before us, and if we turn around, they converge to a point back there too! And yet they appear to be straight and parallel and equidistant throughout their length. This reveals a conformal warp in our very perception of space, such that lines that curve along with the warp are by definition “straight” in that space, and they terminate at (pseudo-) “infinity” at either end. The dome of the sky marks the outer boundary of our perceptual bubble, the maximal extent of our spatial experience, no matter how large a space we are exposed to.
The reason for the warp in our perceptual bubble is clearly to allow a finite spatial representation to depict a practically infinite surrounding space. This is yet another manifestation of closure, with remarkable similarities to the true nature of the number line as revealed by Clifford Algebra.
In my next posting I will show how the similarity with perception does not end there, and that the conformal mapping in Geometric Algebra suggests a similar inversion as a part of perception, except extended out into three dimensions, and the purpose of that inverse representation is the same purpose it serves in mathematics, it reveals certain regularities and symmetries that are not readily apparent in the non-inverse world.
Conclusion
The history of algebra has been the history of an incremental discovery of an evidently pre-existing structure. Clifford Algebra has turned out to be the lynchpin that shows how all the previous discoveries are interrelated within a single self-consistent scheme whose laws operate the same in all directions. It is the very simplicity and generality of Clifford Algebra that confirms its “truth”. But truth to what? What is it that mathematics represents? What must it remain true to? This gets to the question of, as Lakoff and Núñez (2000) called it in the title of their book, “Where Mathematics Comes From“. If algebra is the discovery of a pre-existing structure, then what is that structure and where is it located? Plato and his many modern adherents believe mathematics to be an objective eternal Truth that exists independently of human minds, although it is only accessible through the human mind. It is true that mathematics is not a human invention, but more of a discovery, and thus has objective existence in that sense. But to declare the magnificent edifice of Mathematics to inhabit an orthogonal dimension that is inaccessible to scientific scrutiny, is a hypothesis that cannot in principle be falsified, and thus, it is not a scientific hypothesis but more of a belief, for those who are inclined to believe it. I demand a more scientifically sound falsifiable hypothesis for the ultimate nature of mathematics that gives it objective existence in the universe known to science. I agree with Lakoff and Núñez that mathematics is an artifact of how our mind makes sense of reality, and that therefore mathematics has physical existence or instantiation within the human brain. Our view of the world is not direct, we see the world indirectly, through a representation, or model of the world constructed in our brain, and that model is painted out in the geometric primitives of points and lines and planes, which are the elemental features of perception, and those geometric primitives correspond closely to the algebra which was ultimately derived from them. Since Clifford Algebra offers such a clear view of the essential principles of mathematics, it thereby also offers a clear view of the principles of visual perception and the way we conceptualize shapes in space, revealing algebra to be at the root of it a spatial process of spatial operations that operate on spatial structures. The scalar algebra that we learn first in school is an abstraction of the vector algebra that underlies it.
The periodicity of the number line, the alternation between even and odd, the concepts of negation and inversion, are all strongly suggestive of a phasic cyclic oscillatory phenomenon which must ultimately reside in the brain. The fact that Clifford Algebra reveals all of algebra to be a spatial phenomenon at the root of it, suggests that the computational processes of the human brain are themselves spatial in nature, like the computational operations of nonlinear optics and phase conjugation which they resemble, where spatial waves interact with other waves to produce modulated waves. The fact that Clifford algebra is particularly good at modeling phasic / cyclic phenomena such as the “spin” of elementary particles, all suggests that the human brain operates with a spatial representation based on cyclic oscillations and standing waves in the brain, which just happens to be similar to the cyclic resonances of subatomic particles. The equivalence found in Clifford Algebra between the linear and rotational domains, how negation maps also to rotational reversal, and translation along a linear axis maps to angular rotation about the origin, is surely further evidence for a wave based principle of computation in the brain. Light waves come in the form of transverse waves that oscillate vertically or horizontally, but it also manifests in the form of rotational oscillations whereby the photon spirals either clockwise or counter-clockwise as it travels through space. It is the essential equivalence between linear and rotational oscillations that reflects their equivalence in Clifford Algebra.
The many manifestations of closure seen in different forms throughout algebra suggests a computational principle that makes optimal use of a finite representational resource, like the odometer whose individual dials each form closed loops, as does the odometer as a whole, and thus the mechanism never “runs off the end”, it just wraps around again. This is not a strategy for an accurate representation of an effectively infinite universe, as we discover whenever our odometer “rolls over” to zero, but rather it is a strategy for a wise design of a limited representational resource. It is an artifact of the computational mechanism of our numerical mind with which we represent the world, not a property of the world itself. But there is also a certain invariance to a rotational representation that resolves the problem of the “infinities” found at both ends of every number line. The equivalence between linear and rotational dimensions suggests that our mind never actually uses linear dimensions like the X axis, but approximates them in a circular dimension to avoid the problem of “infinities”.
This entire analysis of Clifford Algebra was based on my own foundational assumption that mathematics is not a human invention, but more of a discovery of the essential principles of computation in the brain. If this hypothesis is correct, then it should be possible to reverse engineer the principles of operation of the brain with guidance from the properties of mathematics as revealed through Clifford Algebra. I propose the next step in investigating the computational principles of perception would be to devise oscillatory circuits such as this Clifford Vectors Analog Electronics Analogy, to see what is possible using waves as a principle of spatial representation and computation. When we discover what kinds of things can be computed using a cyclic / phasic analog system built to emulate Clifford Algebra, only then will we be ready to hypothesize the computational function of the oscillations found in the brain.
This concludes my introduction to Clifford Algebra, but this is by no means the end of the story! This narrative continues with the story of Geometric Algebra, the modern reformulations and some extraordinary extensions to Clifford’s and Grassman’s work, largely due to David Hestenes, that reveal the true potential of Clifford Algebra and its intimate connection with perception. David Hestenes saw fit to rename Clifford Algebra to Geometric Algebra, which it turns out was Clifford’s own choice, because Hestenes wanted to emphasize the fact that Clifford Algebra is not just another algebra, but a radical discovery of the true roots of all algebras, and those roots are geometric in nature.
The story continues with Geometric Algebra: Projective Geometry.
The final chapter is Geometric Algebra: Conformal Geometry.











































































Very good page about geometric algebra..not sure i have seen such an easily digestable account . I just finished reading Tegmark’s new book ” our mathematical universe ” Your words and his are clearly complementary however he posits that the fabric of our universe is in fact a purely mathematical construct and that things like the apparent randomness we see in quantum phenomena are not real but a consequence of this mathematica and how it is perceived by us…he also touches on the problem of the two infinities…. the vanishingly small one called a singularity and the usual big one ( the boundary of our observable universe ). I recommend you read his book and allow it ti stimulate your neurons further..best regards
Interesting counterpoint, (reading the wiki page sufficed) but no different than the Platonic thesis that mathematics is more objectively real than physical reality. This is a paradigmatic choice we all have to make. Whenever we see geometric form constants as in LSD hallucinations there are two alternative explanations: Either we have become magically capable of seeing the true fabric of the universe and that fabric is mathematical, OR we are seeing the fabric of our own brain in its attempt to represent external reality internally, which it does by way of geometrical form constants. One is a magical mystical explanation that suggests that the concrete universe of our experience is merely an illusion, the other is a scientific hypothesis that our brain operates on mathematical/geometrical principles. The former is an unfalsifiable hypothesis, there is no way to prove that the universe is mathematical, the only evidence for it is subjective, and thus that is not a scientific hypothesis, but merely a belief, for those inclined to believe it, it is not something that can be proven to anybody else. The latter is a falsifiable scientific hypothesis that our nervous system “does math” all the time, and it is by that neural mathematics that we can even see the world. I am confident that this will one day be proven by neuroscience, that it is the tendency of the nervous system to spontaneously form standing waves that accounts for visual hallucinations, normal visual perception, and all of mathematics and geometry.
At 76 years and continuing, I have thought as you and assumed it was obvious. So I’m somewhat surprised that such learned minds think otherwise. Our mathematical models remind us that a map is not the territory.
Fascinating… thanks for putting all this together!
You raise a very interesting proposition regarding modelling human computation.
I’m kind of in the middle of reading Michael Arbib’s mirror neuron hypothesis roughly about evolutionary development of language as a symbolic extension of an underlying 3 dimensional rotational mathematics encoded in body movements. Maybe that’s interesting.
Anyway, I enjoyed reading your article, thanks, GA would be ideal for high school. Would have made physics easier…
Very interesting. Will you also conclude the narrative please with a similar visual overview of what David Hestenes has done in Geometric Algebra?
Yes sorry for the delay, I have been working on it but have been distracted by other things, I will try to finish it up as soon as I can.
Pingback: Geometric Algebra: Projective Geometry | slehar
Fascinating. I have come to the same view about how the brain works, but from thinking about chirality and quaternions. Please see http://www.chiralkine.com. Can the maths be reconciled between the two posts?
Hey, thank you for this great article. I’m learning about some of this stuff right now, and it helped very much.
Greets
Pingback: Geometric Algebra: Conformal Geometry | slehar
Very nice article, but a couple corrections need to be made in the Clifford section. The complex numbers and quaternions are isomorphic to the *even subalgebras* of Cl2 and Cl3, not the whole algebras. And the octonions are *not* isomorphic to any Clifford algebra at all! (Easily seen when you realize that the octonions are not associative.) The even subalgebra of Cl4 gets close, but has six elements that square to -1 and one element that squares to +1 instead of the seven elements that square to -1 needed by the octonions. Octonions should also be removed from the figure at the beginning of the article.
Thank you for the errata, I have made the corrections.
there’s a tiny mistake in the picture of quaternion, i^2=j^2=k^2=ijk=-1,
The notion of wrapping around from positive to negative infinity is still bothersome. Presume that true infinity Inf has one location, but is paired with an orientation (a dual of the location). In two dimensions Inf(1) is “positive infinity”, Inf(-1) is “negative infinity”, Inf(i) is “positive imaginary infinity”. That way 5/Inf(3+4i) has a “value” of zero, as does 1/Inf(1). Perhaps there isn’t such thing as an un-oriented zero? If so, does that mean that algebra can be stripped of undefined cases?
or are positive and negative numbers related as enantiomers that emerge from the resolution (in a chemistry sense) of zero due to symmetry breaking (privileging one enantiomer over the other)?
I guess what I am getting at is whether this actually fixes the inverse problem in algebra, or just clarifies why it doesn’t work. assume: Inf(z)*Zero(z)===1, and a*Inf(1)==Inf(a) when a is length 1. It seems obvious that: 10 * Inf(1) * Zero(1) == 10. But 10 * Inf(1) * Zero(-1) == -10. Just blatantly stating a value “0” is ambiguous as “Inf”. But 1/Inf(1) being the origin of the value 0, is not ambiguous – it is Zero(1).
Positive and negative numbers are treated as left, right ordered pairs of numbers [(0, 1) and (1, 0)] that when superposed cancel down to zero. The order in which they are superposed does not matter: the combination is commutative. Suppose this is wrong: that the 1s cannot cancel. Suppose that this is because there is an unambiguous zero that became fixed through the original mental act forming the basis for the numbers. Cancelling the 1s violates a chiral symmetry set by the original mental act. Positive and negative infinities are connected by this chiral symmetry.
Treating positive and negative numbers as ordered left, right pairs of 1s and 0s, when pairs are superposed, the 1s in the same column are added, not the 0s, and the 1s in the same rows are cancelled, not the 0s. 1 is always privileged over 0. Does this overlook a symmetry in which the 0s are added or cancelled, not the 1s. This symmetry connects 1 and 0: it wraps positive and negative infinity around. The choice of which of 1 and 0 is to be privileged (a mental act) sets up the whole algebra. Infinities are a consequence of working in ordered pairs: they are artefacts, because maths based on ordered pairs is not providing the whole picture.
The other alternative, which seems to be implied by this post, is that when there is exactly one value of Zero with no orientation, its inverse is Inf with no orientation (ie: a complex circle around zero with Inf(1) radius). That Inf causes real trouble. If you have an Inf(z) with an orientation, then your Zero(z) … which might properly be called Iota(z), and seems to not create algebraic problems.
The biggest pain in trying to represent an algebra is in having branches (ie “principal branch”) and places where rules such as divide cannot trigger. It should be more like an electronic circuit, where the computation knows the number of (possibly redundant) inputs and outputs there are, and the expression is “branch-free”.
I haven’t absorbed the post on conformal geometry though, which seems to have some of the answers. In any case, Clifford Algebra is already pretty amazing for getting rid of special cases. It just looks like it’s so close to also dealing with the inf/-inf problem that creates difficulty in actually implementing things by creating undefined inputs and outputs.
Pingback: Vector Calculus: Understanding the Cross Product | BetterExplained
Pingback: Is mathematics intrinsic to the Universe? | John Gideon Hartnett
Yes it is. Mathematics is an artifact of harmonic resonance systems. We find it in our brains because our brains operate on a harmonic resonance principle. It is the “magical” properties of resonance that made evolution pick that particular principle for its perceptual and motor computation. See my book http://cns-alumni.bu.edu/~slehar/HRezBook/HRezBook.html
What would be the advantage in using geometric algebra over linear algebra in say neural networks, more specifically convolutional neural nets (time domain or frequency domain) for image recognition? I’ve seen some images of the more basic edge shapes after the initial convolution layer. The standard approach versus the Clifford/geometric approach give different results. This leads then to questions of performance. How fast does one approach train the net versus the other and more importantly how much better a classifier would one approach provide over the other. Also, maybe one approach better mimics what the brain does than the other.
Any thoughts or pointers to work on this?
Pingback: Bookmarks for June 19th | Chris's Digital Detritus
I have been trying to wrap my head around GA for a while now, and this helps me immensely.
As a self-taught mathematics aficionado, do you think it is possible to learn Clifford Algebra / GA directly or differently rather than going through the standard curriculum and then tackling it? I only ask because I am teaching my son mathematics, and would love a ‘gate’ to it without having to unlearn or rethink the scalar, vector way. Thanks!
I believe that ALL of math should be learned “directly and differently” instead of going through the standard curriculum. The most profound implication of Clifford Algebra is that the geometrical intuitions of math are not just a side-effect or coincidence, but that math IS geometry, and that is the way it is best learned. I recommend Geogebra, a fantastic geometry-algebra tool for playing around with mathematical ideas by direct geometrical visualization. I would teach math as “playing with ideas”, and geogebra does exactly that.
I believe that all of math is best taught or learned “differently, rather than going through the standard curriculum”. Geometric Algebra demonstrates that all of mathe IS geometry, our educational system has not yet caught up with that profound reality.
Robert, I’d say spend part of your time studying texts on GA, and part of your time looking up things which it becomes apparent that you need to look at.
For example, you’ll probably find at some point you need a closer look at determinants, so then you could look up lectures on determinants (there are many great ones on youtube).
I’d say keep flicking between different levels of advancedness / abstraction (e.g. vector calculus = low level, tensor calculus = medium, GA = high level).
Keep looking ahead, and keep looking back,
Also, study bits of linear algebra but from different angles – there’s the columns (and rows) and matrices approach, and there’s the more abstract, formal approach. Do a bit of both.
🙂
Clifford Algebra and Linear Algebra produce pretty much the same results, i.e. they are mathematically “isomorphic”, you can translate an expression of one in the other without problem. The difference between them is the mental imagery by which you understand them. Linear algebra is often understood in a purely symbolic way (although the best linear algebra can be demonstrated in spatial figures as in Clifford Algebra) whereas Clifford Algebra is understood as spatial operations on spatial entities.
Pingback: At the edge of reality and dimensionality | Meta Cosmology
Re: psychomathematical hypothesis.
Sounds kind of Kantian 🙂
Thank you both. I sort of do take the ‘look back, look forward’ approach. My momentum some times takes a hit when I realize there is a basic hole in some of my self-taught math, and I have to rally to fill in the hole and move on. There is something about GA that just works for me from an intuitive, or geometry angle. I have used Geogebra, and I sometimes use AutoCAD to layout some ideas when I cannot hold the full geometric concept in my head. For me, GA holds the promise of concise notation for my math journal, along with the geometric, intuitive approach, so that I can go back and look at my entries and the density of information in the GA forms will allow me to put a lot on one page. Thanks, again!
Rob
Brilliant. My son has just started doing things with Clifford Algebra and this has allowed me to have at least some understanding of what it is, and why it is so useful for what he is doing.
Thanks very much for this – clearly a *lot* of work, and well done!
I’ve been looking for a better place to send readers of my research – see TauQuernions.org – which uses a Zed3 = {0,1,-1} geometric algebra to describe asynchronous distributed computations. For example, a+b states that the 1-bit processes a,b are concurrent; their co-boundary ab is a two-process spinor; etc. This gives a very geometric (read spatial) account of distributed computation, including QM.
I’ve also applied this GA process encoding to AI, and am in complete agreement with your thoughts re resonance and mind – see RootsOfUnity.org (unfinished and unpublic, but full of hope).
The Great Connector in all of this, which seems to have gone largely unnoticed (silo effect), is Parseval’s Identity, the generalization of the Pythagorean theorem to n dimensions. This connects Fourier’s world to that of discrete points, whence sums of sines and cosines equal sums of squares sqrt’ed . The Identity applies because in geometric algebra, all of the 1-vector generators {a,b,c,…}, and their products {ab, ac, …, abc, …}, are – precisely – mutually orthogonal. After almost twenty years of working with Clifford algebras, the 2011 realization of this connection lit me up, recalling Emily Dickinson’s lines “The Truth must dazzle gradually, or every man be blind”.
This is a very different use of GA, but I hope you’ll like it anyway.
Excellent intro! However, I’m a bit confused by your treatment of pseudoscalars (which you seem to define as trivectors) and their relationship to the imaginary number i. Specifically, what does “I**2 = -1” mean? The asterisk is previously used as a multiplication symbol, but here it seems to be used as a double-exponent in front of what could be a numerical exponent.
The “imaginary number” i is severely mis-named. It is not “imaginary” but “Rotational”. A multiplication in a closed space where if you go far enough you wind up right back where you started.
Multiply by i and you get quarter-way round. Multiply again by i and you wind up pointing in the opposite direction to where you started.
Likewise with the pseudo-scalar: Multiply by I (= e1^e2^e3) and you turn quarter-circle in all 3 dimensions. Do it again (multiply by I twice in succession) and you are facing backwards in all 3 dimensions relative to where you started.
A question about the ‘Geometric Product’ section.
“Note that a dot product between two scalars produces zero, because neither has any spatial extent and therefore they have no projection on each other, and thus the scalar multiplication with which we are all familiar is actually a wedge product.”
Is this correct? Following the logic, the dot product between to scalars would be regular multiplication and the wedge product would be zero.
Scalar multiplication of a vector would also still be the dot product as the scalar commutes .
I puzzled a long time about the dot and wedge “products”, in what sense were they both “multiplications” because it seemed to me that the wedge product is “multiplicative” in the ordinary sense of the word, i.e. it produces a multiplicity of its multiplicands, it is an expansive process that expands outward from the given operands, whereas the dot product is more of a collapsing projection, like an object collapsing to the shadow it projects. This seems more akin to division, in the sense that it reduces its operand to a lower dimensional projection. Seen in this light, the standard scalar multiplication we first learned in school is a multiplicative expansive operation, we were never taught the dot product of scalars, because it is always zero, which is not very useful.
The “standard scalar multiplication we first learned in school” is a commutative operation, however the wedge product is NOT a commutative operation, (its anti-commutative A ^ B = – (B^A) ) and therefore they can not be identified. The dot product of two scalars equals the geometric product of the scalars, which equals the “standard multiplication”. The wedge product of two scalars is zero. Let a and b be scalars, and a ^ b their wedge product. Since scalars commute with the entire algebra and b^b = 0 (for any blade), a ^ b = (a.1) ^ (b.1) = (ab)(1^1) = 0. So the dot product of two scalars is the same as the “standard multiplication”.
Goodness! I think you are right! I will have to correct that. Thanks for paying attention, I could have been spouting that un-truth for years but for your correction! (I just discovered Clifford Algebra a couple of years ago and am still learning!)
When explaining the geometric product, you write in the section on Clifford “A vector times a trivector […] collapses back down to a scalar, thus maintaining closure in three dimensions”. But multiplying a vector with a trivector produces a bivector (e1e123=e23). Multiplying a bivector with a trivector produces a vector (e12e123=-e3). This is the principle of duality. Unless you are referring to the outer product, in which case e1 \wedge e123 = e12 \wedge e123 = 0. Similarly, when explaining the exterior product, you write in the section on Grassman “closed: a \wedge b \wedge c \wedge d = 0”. I’d rather call that the principle of annihilation. That is why the composition of the geometric product of an inner and an outer product is so useful. One preserves, what the other annihilates.
Closure, understood as “wrapping to scalars” (not just to 0), can occur in various situations: when multiplying two linearly dependent trivectors (e123e123=-1), two linearly dependent bivectors (e12e12=-1), two linearly dependent vectors (e1e1=1), or two scalars. Or, to put it differently: When two geometric objects are so similar, that their relationship can be expressed simply in terms of “scale”.
I propose to express closure in terms of degrees of freedom. In 2D there is one degree of freedom for scalars, two degrees of freedom for vectors, one degree of freedom for bivectors. In 3D there is one degree of freedom for scalars, three degrees of freedom for vectors, three degrees of freedom for bivectors, one degree of freedom for trivectors. In 4D there is one degree of freedom for scalars, four degrees of freedom for vectors, six degrees degrees of freedom for bivectors, four degrees of freedom for trivectors, one degree of freedom for quadrivectors. And so on… As the dimensionality of the geometric objects increase, the degrees of freedom first increase, then “wrap around” at a maximum value, and decrease until reaching 1 again; closure. “abcd=0” would leave no degrees of freedom, at all.
Having said that, I believe that your presentation on Clifford algebra is one of the best around. Great work!
Thanks for the clarification, you seem to have a deeper understanding of Clifford Algebra than I do (I only discovered it recently and am still learning). I will try to incorporate your suggestions. Thanks for your appreciation!
No, “the real and imaginary dimensions operate by different rules. … but this is VERY STRANGE!”, is not strange — the Linear-Cyclic or Male-Female duality pattern is THE fundamental building block of nature of which mathematics (primes) is but one example. This is pattern is pretty common knowledge in Sacred Geometry and with Mystics. This pattern keeps getting re-discovered; Johann Wolfgang von Goethe wrote it eloquently as “Music is liquid architecture; Architecture is frozen music.”, the modern vernacular paraphrases this as “Geometry is Frozen Music”, meaning everything in the physical universe is based on Frequency.
This was a Python implementation of a general multivector that only uses orthogonal vectors:
https://github.com/rfielding/gaMul
The type of the vector depends on which entries are exactly zero (which becomes a little fuzzy in the presence of floating point arithmetic). I had never seen such a definition stated elsewhere (but kind of hinted at in a book that I have). I couldn’t figure out how to define it to handle non-orthogonal bases such as 5d conformal geometry. Also note that this is also O(2^n) with the number of dimensions in use, but should be of use for low dimensions.
Fascinating, and very helpful. I will mention this article in the LinkedIn group that I formed for preparing instructional materials for GA: https://www.linkedin.com/groups/8278281.
Please note that I am by no means an expert in GA: I formed the group as a space for collaboration among GA users and the high-school teachers who will need to learn to teach GA, as well as how to solve problems with it.
Along those lines, I will welcome comments and criticisms of materials that I’ve developed for other self-learners. Links are given in posts to the above-mentioned LinkedIn group.
great!
I just read your intro article on Clifford algebra. I’m not particularly adept at math but I’ve always been interested in philosophy. I’m currently struggling through Roger Penrose’s book The Road to Reality. I’m only 20% done with it, but it’s not helping as much as I’d like to make a connection between math and some of the philosophical ideas I’m used to reasing about. So I’ve been googling to find supplemental info that’s a bit more “user friendly.” Which led me to your site. Your article was *amazingly helpful*, and I can’t wait to read the other articles that discuss how this algebra relates to human perception. I’ve bookmarked your site as it looks like a treasure trove! Your ideas are very thought-provoking, and your writing style and visual aids are a great help for a non-expert such as I to begin grasping this stuff.
Thank you for your kind comments! Yes the philosophy of mathematics is fascinating. Check out my Perceptual Origins of Mathematics for a totally visual explanation of the true nature of math, which is mental imagery, all explained in pictures without equations. Work in progress – I am still working on it.
Hey! Either you give our Hermann Grassmann back all of his n’s or we will steal one f from your Cliford ! (You could keep one r from Hermann, though 😉
Thank you for the time spent on all the illustrations, that helped me a lot.
Kind regards from Germany! 🙂
P.S. He actually would have spelt himself Graßmann, but we are not asking the impossible.
Vielen Dank für Ihre Aufmerksamkeit!
Die fehlenden Eßen und Ennen sind korrigiert. Für so ein würdige Mathmatiker soll ich mindenstens seinen Namen richtig buchstabieren. Und danke auch fur Ihre Anerkennung.
Grüeße aus Amerika!
Isn’t saying “The truth, or validity of Clifford Algebra is confirmed by Occam’s Razor” akin to saying “The truth, or validity of Linked List is confirmed by Occam’s Razor”?
Saying things like “Clifford Algebra is true” doesn’t really make sense, because Clifford Algebra is not really a theory or system of belief or proposition. Clifford Algebra is more like a particular data struct, and _a_ Clifford Algebra is like an instance of that struct.
It all depends on what you believe mathematics IS. Whether you believe mathematics is a human invention, like a Linked List, or whether you believe mathematics is hard-wired in the human brain that is reflected in math. Advances in mathematics are not human inventions, they are discoveries of the foundational principles of mathematics, which in turn reveal the foundational principles of human cognition. Clifford Algebra is “True” in the sense that it has captured the essential computational and representational principles of the human brain. But not everybody believes that that is what math is all about.
This is all very interesting and I want to ask a very naïve question: In my old age I want to learn about Neural Networks and Deep Learning but my maths is limited to Maths 101 at uni many decades ago – is it possible that using Clifford Algebra in a visual way could help see what is going on with NNs etc? – in particular I find Back Propagation confusing . .
Thanks,
Phil.
You don’t need Clifford Algebra to understand neural networks, but the idea of understanding things visually certainly does help. I too was confused by the secret behind neural networks, so I set out to understand it visually. I wrote a program that displays the input, output, and hidden layers of a 3-layer backprop network, and watched the pattern of weights in the hidden layer change as I trained it on some patterns. The results were very illuminating.
When training on an input pattern of, say, 1 0 1 0 1 1 0 0, it would create a pattern of columns in the hidden layer aligned with the input pattern.
1 0 1 0 1 1 0 0
1 0 1 0 1 1 0 0
1 0 1 0 1 1 0 0
1 0 1 0 1 1 0 0
1 0 1 0 1 1 0 0
There was also a pattern of rows that matched with the training pattern. For example if the training pattern was 0 0 0 0 1 1 1 1 (imagine this as a vertical vector along one side of the hidden layer image) it would produce four bright rows along the bottom (for this example) that meshed with the column pattern, and suddenly I could see how the whole thing worked.
The more general lesson is that ANYTHING mathematical is most easily learned by “seeing the picture” in your mind. Then math transforms into a beautiful intuitive thing that is a true pleasure to learn!
Check out my other WordPress site (work in progress…) on
The Perceptual Origins of Mathematics”
It paints a completely different picture of what math is all about, I think you will enjoy it. It is never too late to learn math, but it sure helps to learn it the easy way, which is through mental imagery. See also
Better Explained
a web site that explains all those things that always confused you, in a simple intuitive way, that will make you ask “Why the heck didn’t they teach it that way?” It is a serious problem in math education.
I see you are also a fan of Paul Lockhart ! One of greatest modern crimes is teaching Mathematics by rote instead of (self) discovery 😦
A Mathematician’s Lament
Click to access LockhartsLament.pdf
Wow! thank you for such a fast and very useful response! – I really appreciate it! I think you have pointed me in exactly the direction I was looking for. For the record, I have the same question (“Why the heck didn’t they teach it that way?”) about Music Theory – and I am still looking for a teacher with a brilliant way of making sense of most of that field . .
On music theory, I would recommend Garage Band on the Mac. (Are you a Mac user?) They have a music composition function that can either display traditional staves and crotchets (I have always been bitching about that representation!) or alternatively, they have this great visual display of music that looks like the music sounds! Notes are little rectangles that you can move around with point-and-click, they make their sound as you move them around, you can select, copy, and paste whole sections of notes. It takes a while to get used to the user interface, but I have written some lovely little dittys and had a lot of fun with it.
I am a Linux user but I have an iMac VM – but I have never used it for producing sound . .
We are on the same page re Traditional Notation: http://philiprhoades.org/music/gnote2_1.pdf
What language did you use for your NN/BP exercise? Would you share it?
BTW, I have signed up with Better Explained and left a note for Kalid re NNs.
Geometric Algebra/Calculus is awesome, but I strongly disagree on the philosophy of math here. Clifford algebra is useful as a representation of physics because the rotational symmetries of physics (which are completely independent of our minds, of course), are what sets its structure. As you note, many other formalisms are isomorphic, and if you have been through developments of physics in those formalisms, you see that you get to the same place by imposing those symmetries. A favorite example of mine is comparing the standard linear-algebra approach to developing Lorentz transformations to Hestenes’ Spacetime algebra (STA) approach (i.e. the clifford algebra in 4 dimensions where one of the basis vectors e0 has magnitude squared e0e0 = -1). The only reason the STA approach is superior is that those constraints are inherent in the algebra because of the way it’s defined, whereas they have to be imposed after the fact in the general linear algebra version.
But in the end, either algebra is just a set of symbols on a page – they have their structures independent of the minds that conceive them, and independent of the world. The theories can be used to describe the world because the world has the same structure. (I similarly don’t think there’s any paradox in mapping infinite domains into finite ones – as long as the math doesn’t generate any contradictions (and it provably doesn’t), there’s no reason this should be impossible. Whether it’s physical is a separate question). We don’t see periodicity because our brains have periodicity but because rotations just are periodic.
There is a connection to minds, but I think you have the wrong one – I think our minds have representations that are described efficiently by GA because our minds are adapted to a world that is described efficiently by GA. Note that physics isn’t made of math, either, on this view; it’s just isomorphic to math. Isomorphism is the key really — there’s nothing mysterious going on.
Well I guess I strongly disagree with your strong disagreement. There is something very mysterious going on! Yes all those algebras are isomorphic, but that is exactly the point – isomorphic with what? With reality itself? Thats what you believe. Isomorphic with the way our mind makes sense of reality, thats what I believe. Check out this paper on the biological implications of all this:
Double Conformal Mapping
But then I suspect you won’t agree with that one either. The deep mystery is what makes it interesting! You are missing the best part of the story!
You’re right that I still don’t agree. I think you’ve missed my point. Isomorphism is an equivalence relation, so if visual perception is isomorphic to some algebra, then that algebra is isomorphic to visual perception. Isomorphism doesn’t have a direction, and has no bearing on whether the theory is math. Whether or not they actually are isomorphic is a scientific question (might even be interesting, as you suggest, but entirely beside the philosophy of math point). I’m not saying you have the direction wrong, that actually the physical world is what’s relevant. I’m saying that the isomorphisms to stuff in the world are a completely separate issue from the math; they’re necessary for applying math, but you can write down the theory either way.
There are plenty of other areas of math out there, say topology, number theory, probability, category theory, to name a few broad fields. You seem to say that mathematics is about discovering the structure of our mental representations. Does that mean category theory is not ‘true’ math? If so, then what you mean by math is not what anyone else means by math.
And furthermore we can write down plenty of perfectly self-consistent geometrical theory that doesn’t necessarily correspond to either the physical world or to perception. You present some theory about some particular projections that you think match our visual perception, but you could easily write other projections (say, replace the sphere with an ellipsoid). And at most one of them would truly represent perception. Would you say the other one wasn’t ‘true’?
It makes much more sense to think of all the theories as ‘true’ math, and say that the question you’re interested in is a scientific question about what math describes the structure of your thoughts, rather than being in any sense about which math is ‘true’.
Thomas Shaw, In your reply (below slehar’s reply) you say “Isomorphism doesn’t have a direction”.
To me this isomorphism looks like the deeper underlaying problem of explaining, or defining, information (shannon information aside in this case);
One could say that information needs an interpretor (or, must be possible in principle, to interpret) in order to be information (meaning, acctually transfering information).
Or in other words, it’s a chicken&egg problem; Which one comes first? The chicken (the material universe) or the egg (the math) which is describing (watch out for the paradox) what potentially already exist.
So yes, I think you missed the “mystery”. I say “mystery” only because whether it’s a mystery must depend on your axiom, namely how everything came into existence in the irst place.
In short, cosmogony. Which is, it follows logically (due to our axioms), essentially a religious question.
The sender-receiver (chicken&egg) structure points to intent. One cannot exist without the other. It simply can’t.
// Rolf Lampa
For whatever reason, the Cartesian anxiety hyperlink didn’t go through; it was supposed to be to the 2016 edition of “Embodied Mind: Cognitive Science and Human Experience:
https://mitpress.mit.edu/books/embodied-mind-0
If multiplication with i do rotation, what means pi and e constants, or they exists only for calculation in terms of Cartesian coordinate system, angles etc?
Rotation is preferably via a double reflection through the half-angle between two example vectors in GA, because there is more to it than 2D and 3D space. You can use pi, e, sin, cos, etc if you want; but you are not required to use them to do the geometry.
Take a look as well at this, Euler’s formula via introductory group theory:
Oops! Help, please, Steven.
Hey Michael Manthey, that is a beautiful presentation! My favorite kind of math, expressed as visual imagery. I believe that is the “real” math. In the most general terms, Euler’s formula relates an affine space with a vector space, and shows how rotation about the origin can be equivalent to flipping sign and reflecting about it. Even more generally, this relates the “egocentric ‘dartboard’ polar coordinate system” (vector space) with the “allocentric ‘city-block’ cartesian coordinate system”. Thanks for the great visualization!
Two things:
1. Yes, it’s a great video, but it’s [unfortunately] not me! It’s from 3Blue1Brown.com
2. You can make neat computer vision algorithm with tauquernions.
-m
Turing would have loved this presentation: the use of math to enable the mind to understand itself and thus nature.
Pingback: Taking ‘brain waves’ seriously: Resonance Theory | Opentheory.net
In the section on Josiah Willard Gibbs you list some of the reasons why the cross product is a big mistake — I would add to the list: The vector cross product is NOT ASSOCIATIVE either.
(AxB)xC does not, in general, equal Ax(BxC). The lack of associativity can be seen from the connection of the wedge product (which is associative) and the cross product:
AxB = *(A^B) and so you get into trouble with triple cross products
(AxB)xC = *( *[A^B] ^ C)
Ax(BxC) = *( A ^ *[B^C])
and since the right-hand-sides are not, in general, equal the cross product is not an associative product.
That seems rather unnatural to me.
Yours, John Smith
Thank you for this post – it was a very insightful read and I think visual mathematics does so much justice to this mathematics of the future. I am deeply aligned with your opinion that the purest forms of mathematics are a discovery, not an invention. I also agree that mathematics can give insight to how our brains work. However, as pointed out in a previous post, you seem to downplay a bit the fact that it is the physical universe, not our brains, that is teaching us which mathematical discoveries are important, and which ones are just symbols on a page. The algebras you discuss have relevance precisely because they provide simpler representations of the physical world than other mathematical models we have used, and they show a level of connected-ness that cannot be ascertained without their framework (such as collapsing Maxwell’s equations into one). And because our brains are made of the same physical stuff as the rest of the universe, it makes intuitive sense that a better mathematical model of the physical universe can also be used to create a better mathematical model of how our brains work.
To get on my soapbox for a second – the fact that mathematics works to describe our physical universe tells us: (1) that the physical universe is logically consistent (for the most part); and (2) that there are symmetries in the physical universe (as stated in a previous post), which we know exist up to a certain level of accuracy.
When nature approaches a logical inconsistency (such as 1/x), there must be a way to get around that inconsistency; e.g., by shooting out a photon of light. If there was an observable logical inconsistency in nature, it would lead to paradoxes that we probably could not wrap our heads around, and almost certainly wouldn’t be able to use mathematics to describe. That the universe is logically consistent even in the infinite spaces we don’t yet understand is a matter of philosophy at this time.
The fact that there are symmetries to the physical universe up to a certain level of accuracy is evident by the fact that we can write equations at all that say “this equals that.” Without symmetry, we wouldn’t have nature’s building blocks, and the simplest of structures would require too much energy to create. But we wouldn’t have known without studying the physical world that these symmetries existed, and if these symmetries didn’t exist then none of this beautiful algebra would be useful. That the universe is symmetric below the level of accuracy we can measure is, again, a matter of philosophy at this time.
On a side note – I don’t share your dis-satisfaction of the cross-product (and the fact that it is non-associative as pointed out by the previous post). These are simply limitations of our views of vectors. As Clifford and Geometric Algebra bring to light, but which we still haven’t been able to fully internalize because our formal education gets in the way, is that we have to stop thinking about vectors and scalars by themselves. When you think of vectors and scalars together, the cross-product is much more beautiful then your writings suggest. Plus, I always get a little weary when someone claims that a mathematical structure that clearly has tremendous applicability to physics does not have deep meaning behind it.
I’m going to be writing a book in the near future on a mathematical framework that shows many of these algebraic ideas in a framework that I think is much more intuitive and easy to understand. So easy, in fact, that I plan on teaching it to my three young kids when they get to middle school. This is based on 15 years of my personal self-reflections (and rotations) on this topic. I was a physics and math undergraduate and went on to get an M.S. in mathematics – even though I work as an engineer for my day job, I have continued to advance this work in my spare time. I did all this in a vacuum, only to become aware more recently of Clifford and Geometric Algebras as I started doing research to support a Ph.D. thesis on this topic that I will be pursuing starting next year. I think these previous algebras are good starting points, but they are missing some basic principles that make them more complex and less applicable than they should be. If you are interested in talking about this more let me know – I have been struggling to find anybody I can share this with and based on work you’ve done I feel like you have a deep appreciation for the beauty and connected-ness of this type of mathematics.
Reblogged this on Tianchi Chen's Blog and commented:
nice intro to clifford algebra
Thanks for appreciating! Perhaps you might like this one too?
https://doubleconformal.wordpress.com/
I love to play at the interface between math, perception, and mind. There is some fruitful stuff to be discovered around there!
Right before “For example Clifford Algebra reduces Maxwell’s equations of electromagnetism to a” you show Maxwell’s equations in vector and GA forms, but use a 1=epsilon_0=mu_0 form with vectors, and SI units with the GA form. Suggest using SI on both sides for a better comparison.
I left a comment on your Quora answer, the one which links to above, and I would leave one here as well! You might find the following physics papers of interest; read in isolation they are, perhaps, not so profound, but, read like chapters in a book, they become rather interesting (one is by David Hestenes):
Now, and the Flow of Time, by Richard A. Muller and Shaun Maguire
Cosmology from quantum potential, by Ahmed Farag Ali, Saurya Das
Electron time, mass and zitter, by David Hestenes
Some Initial Comparisons Between the Russian Research on “The Nature of Torsion” and the Tiller Model of “Psychoenergetic Science”: Part I, by William Tiller.
These papers should be especially interesting if you first read the experimental results I linked to in the comment on your Quora answer! You also may be interested in some original mathematics I completed this last summer. Almost a year ago I invented a counter-example to Tennenbaum’s Theorem. I established set theoretic foundations in ZFC/AFA but essentially you can think of it as the structure {N x N, <, +, *, (1,0), (0,0)} with the lexicographic order, coordinate-wise addition, and multiplication defined by:
(a, b) * (c, d) = (a * c, b * c + a * d + b * d).
It’s pretty straightforward and simple to demonstrate that these operations are recursive and, of course, there is no isomorphism, hence, the counter-example.
After writing a short paper formally demonstrating the counter-example, I immediately embarked on extending it out to algebraic closure and, in the process, realized (discovered) that there exists a countable subsumption hierarchy of Universes with recursive functions which conforms to the geometric sequence {2^n}; the Universe we traditionally call “standard” is simply the zeroeth-order Universe in this hierarchy! We have:
0) {N, <, +, *, 1, 0};
1) {N x N, <, +, *, (1, 0), (0, 0)};
2) {N x N x N x N, <, +, *, (1, 0, 0, 0), (0, 0, 0, 0)};
3) {N x N x N x N x N x N x N x N, <, +, *, (1, 0, 0, 0,0, 0, 0, 0 ), (0, 0, 0, 0, 0, 0, 0, 0)};
.
.
.
I have very little formal education and no affiliation so I posted the relevant papers to Vixra:
http://vixra.org/author/wes_hansen
In the Q-Universe paper, the order as defined on the q-rationals is not satisfactory in that it doesn't hold on the entire set, but I tried several different things and couldn't seem to get anything to work satisfactorily. So I went with the standard definition because it holds over most of the set and I could at least prove well-definedness!
This is the clearest exposition of the concepts underlying Clifford Algebra that I have found. I am working through John Snygg’s text on differential geometry using Clifford’s algebra, which is also very good but your page has lifted multiple veils from my eyes! I have been convinced for a long time that progress in physics is being slowed by the rather disparate and unnecessarily complex mathematical approaches used in different sub-disciplines. Kudos…..
My answer to the question: “If geometric algebra is so good, why is it that 140 years after William
Kingdon Clifford’s death it is still not universally taught and used?”
Each Clifford geometric algebra provides a general vector analysis for a geometry of a higher
dimensional vector space. Probably the simplest way to introduce them to the widest possible audience is to directly relate them to the most familiar mathematical object that fully captures their essence. By a geometric matrix algebra I mean a real or complex matrix algebra of order 2^n x 2^n. The simplest case, when n=1, is for 2×2 real or complex matrices. The next case, when n=2, is for 4×4 real and complex matrices. Once the reader understands these two important special cases, which cover the important geometric algebras of 3 dimensional Euclidean space and the 4 dimensional Dirac algebra of spacetime, referred to by Hestenes as spacetime algebra, all other geometric algebras can be constructed by taking Kronecker products of successive copies of the 2×2 real and complex geometric matrices. This approach to geometric algebra is systematically explored in my new book, “Matrix Gateway to Geometric Algebra, Spacetime and Spinors”,
https://www.amazon.com/s?k=Garret+Sobczyk&ref=nb_sb_noss_2
A short 2 page introduction to geometric matrices, of orders 2×2 and 4×4, is provided in the Appendix of my article, “Notes on Plucker’s relations in geometric algebra”, to appear in Advances in Mathematics 363, 106959, March 2020. Elsevier has kindly provided a temporary free access link to this article, which can be found at the link:
https://www.garretstar.com/secciones/publications/publications.html
Garret Sobczyk
https://www.garretstar.com
January 30, 2020
Q. Why is it that 140 years after William Kingdon Clifford’s death it is still not universally taught and used?
A. Many reasons:
a) “Science advances one funeral at a time.” — Max Planck.
b) Too many teachers don’t know, or don’t care.
c) “Good enough” is the enemy of better.
Yes, all of that is right. But there is one more factor: math has a dual nature, the analytical concepts, and the symbols by which we represent them on the page. Academia, especial in its pedagogical function, strongly favors the symbolic manipulations over the intuitive spatial concepts, because the former is much easier to quantify in tests and exams, the latter is kind of nebulous – its hard to tell who really understands, and who merely memorized the material. We need a revolution in the way we teach math in school!
I think the physicists played a big role by focusing so exclusively on Lie algebras, which in the present context can be viewed as emasculated Clifford algebras, in that the Lie algebra’s commutator product [XY-YX] neatly discards all reversible interactions. That’s a lot to throw away! In my view, in so doing they very clearly threw the baby out with the bathwater, and as well stunted development until Hestenes in the 1960’s revived it.
[This corrects my preceding comment (unless Steven has deleted it per request)]
I think the physicists played a big role by focusing so exclusively on Lie algebras, which in the present context can be viewed as emasculated Clifford algebras, in that the Lie algebra’s commutator product [XY-YX] neatly discards all commutative interactions. That’s a lot to throw away! In my view, in so doing they very clearly threw the baby out with the bathwater, and as well stunted development until Hestenes in the 1960’s revived it.
Furthermore, the prominent American scientist Josiah Willard Gibbs (1839-1903) took Hamilton’s / Clifford’s anti-commutative product (a)(b) and converted the resulting 2-vector into a 1-vector by mapping its 2d orientation into a 1d vector. Anybody who has learned vector algebra in a physics or engineering course in the years since Gibbs knows his work: “ab” became the vector cross-product axb = c, where c is a third orthogonal dimension. Gibbs did this simplification so that ordinary electrical engineers could more easily avail themselves of Maxwell’s equations. After all, one really only needed just these three dimensions … which thus banished all other dimensionalities from mind for almost a century. Then, as the intricacies and mysteries of quantum mechanics rolled in, physicists began defining a zoo of specialized algebras to recover what had been lost from view. Enter Donald Hestenes. [And meanwhile, the mathematicians had long ago filed Clifford algebras away on a dusty back shelf as an algebra of ‘ideals’, which totally obscures what most folks reading this blog find most interesting.]
Finally, it didn’t help that Hamilton himself pursued the interpretation that ab operating on b, (ab)(b) –> a, could be understood as transforming b into a, which never led anywhere.
Thank you for the clarification.
Can you please elaborate on what you mean by: “[XY-YX] neatly discards all commutative interactions.”
would be appreciated. Thanks!
Cuz if X and Y commute then XY-YX = XY-XY, so the product is zero.
this is a fantastic article. Thank you and keep it coming!
Thanks for your appreciation!